Table of Contents
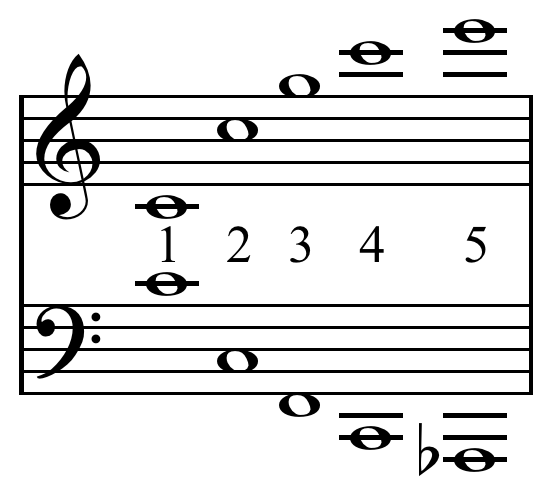
The Undertone series, also commonly called the subharmonic series is a series of pitches with mathematical relationships to one another that are a naturally occurring sequence the can be represented by divisions or multiplications of equal measured parts. The series is an inversion of the overtone series, which can be described through equal divisions of string length, the undertone series can be described by equal additions of string length.
Methods to play an undertone series
Acute bowed force, as to interrupt the natural vibration of a material
On strings
This is only possible on instruments with large wound strings that are very long such as the cello and double bass. The method involves bowing the string strongly, in such a manner that causes the string to vibrate as though it had a longer overall length than is typical, shifting pitch following the undertone series as the firmness of the bowing is increased. This type of bowing should be considered a form of regulation. Typically the three notes in the series can be generated on a bowed contrabass
on Lamells
The only example of this phenomena can be seen on is the terpodion, which is bowed lamellaphone that bows tongues with large flat pads at the ends on each tongue. The tongues are bowed against a rosined cylinder by the force of the tongues attempting to return to a resting position which is at tension against the cylinder, and when the cylinder spins the tongues are moved transversely and repeat the process based off the degree of mass at the end of the tongue. Much like the action of forced vibration on corrugated surfaces these tongues may not always return to position at the resonate pitch, and with the correct regulation the tongues can play an undertone or an overtone of the original pitch.

Forced vibration instruments
On instruments that use forced vibration or corrugation the undertone series can be produced by allowing a plectrum to 'skip' notches of a corrugated material. There are two ways to do this, the first is to increase the speed the plectrum goes over the ridges, in which case there is a point where the plectrum will start to skip ridges because it does not have enough time to return to resting position in between tapping the corrugation. The other method is to hold the plectrum very softly against the notches as to allow the plectrum to only very slightly tap them. In both cases the pitch generated goes down following the undertone series but can be substantially be out of tune depending on how many notches are present and how well they subdivide mathematically as compared to the subharmonic series. This maneuver is most easily done on the Savarts wheel.
Reduction of air on invert reeds
Brass instruments can be 'underblown' by the reduction of air into the instrument paired with a loose embouchure. This technique is most commonly possible on instruments such as the didgeridoo, contrabass trombone and tuba. This series also follows the typical sequence of the undertone series, but may be somewhat out of tune as the pitches are lowered. On a didgeridoo of reasonable length the first 8 pitches in the undertone sequence can be generated.
Selective venting of wind instruments
Long wind instruments such as the bassoon and contrabass clarinet have the ability to play subharmonics using an unusual phenomena of selectively venting small air holes at key locations along their standing waves. this is most commonly done on the bassoon and contrabassoon by venting the low Bb (all keys closed) and allowing a small amount of air to escape the hole for the mid-range A, which results in an A one octave below the one being vented (the A just below low Bb). With an exceptionally skilled player this technique can be used to vent several pitches in the mid range allowing for a low A down to Eb to be played. this technique is easier to produce on reeds that easily speak low notes and pairs well with a very loose embouchure. On the contrabassoon up to three notes of the undertone sequence on the vented A have been documented. A similar technique can also be done on the contrabass clarinet. In both cases the sound of the generated notes is rough and the technique is exceptionally fickle and difficult to produce, and does not represent an easily repeatable, reliable, or fast technique for compositional purposes. This kind of selective venting may be possible on other instruments as well and under well constructed circumstances may be able to play many notes of the undertone sequence.
Extension of length
Just as the overtone series can be generated from equal divisions of a length– for example dividing a strings length by half, thirds, quarters, fifths and so on… The same sequence can be reversed and the length is increased from an original length by the same degrees, generating an undertone series by doubling, tripling, or quadrupling (and so forth) the original length of a string. The same can be done by dividing the length of a tube (possible by the addition of holes as well) and can be done in the reverse by increasing the length of the tube (buy covering holes as well) all by equal divisions of multiplications of the original length. This method of generating the undertone series should not be viewed as a pure form of the series and does not fully represent the phenomena in the natural manner it occurs in the other examples listed above, this is more of a mathematical modeling that leads of pitches that are in common with the natural phenomena, though from a sound generating position they are both the same.
Notes
All of these techniques are only producible on large instruments. The highest starting pitch that is capable of generating this series is on the Terpodion, which is under high resistance with fast spinning, representing a degree of force difficult to make on most instruments that are directly played by a player. The undertone sequence has great potential to allow for instruments to play far lower pitches than are commonly available for a given length of instrument. I wonder if there is a relationship to stopped pipes and the undertone series (a cylinder like a clarinet is 1 octave lower than a conical instrument of the same length) and this may represent the first harmonic of the series. It is also ambiguous how the octave above a fundamental relates to the octave below it and now those relate to both the undertone and overtone series (when moving from the undertone series to the harmonic series is is there a total of 4 octaves or 3 octaves in a row when shifting from one series to another… or perhaps its 2, with the fundamental of each series being the first harmonic of the other).
Harry Partch described it as 4 octaves in total in his theory of Otonality and Utonality